March 24: District Assessment

DON'T FORGET CONFERENCES ARE THURSDAY, MARCH 26!

Today we took the District Assessment, and that's about it!  If you missed it, please be sure to make that up after break!

Speaking of break, here's a fun fact for you:

No, seriously.  True story :-)

SEE YOU IN APRIL!!!

March 23: Review for District Assessment

Since this week is only 2 days long, there's no new learning, so no new notes!

We have our 3rd Quarter District Assessment on Tuesday, March 24, so Monday we will be reviewing for that.  And remember, the District Assessment does NOT count toward your grade- it is ONLY used by the District Office to determine how our new curriculum is working!  So don't get too stressed about it, but still try your best! 

Click here for the review activity.

March 20: UNIT 7 TEST

Today we took the Unit 7 Test.  I will pass them back on Monday, and then you have until Tuesday to make any corrections you choose to make. 

LAST DAY OF THE QUARTER IS NEXT TUESDAY, MARCH 24!

March 19: Review for Unit 7 Test

I was gone today (darn migraines!) so the guest teacher passed out an activity for the class to do.  The problem was that she passed out the wrong activity!  Since it was my mistake (I know, I was as shocked as you that I made a mistake this year!) there was no homework tonight. 

Unit 7 test TOMORROW on Pythagorean Theorem, converse of Pythagorean Theorem, distance formula, and finding distance on a graph.

You may use your notebook on the test, but be sure to study so that you don't use your notebook as a crutch!

Have a great day, and see you tomorrow!

March 18: Map Activity

Today we worked on an activity that requires Pythagorean Theorem to solve a real world problem.

Students were given a map of a neighborhood in Spokane, and the premise is that a new Cable Company has opened and needs to run fiber-optic cable to provide service to the area.  The map has several diagonal segments connected at street corners, and students are to use Pythagorean Theorem to determine the lenghts of each segment of cable.  We did the first one together in class, and everyone seemed to pick up on the activity quickly.

If you were absent today, please make an effort to complete the activity using the example triangle given for #1.  If you have any questions, please be sure to come in during Advisory for help!

Click here for tonight's homework.

March 17: Applicaiton of Pythagorean Theorem

Today we discussed when Pythagorean Theorem can actually be used in the real world.  We had several examples of real-world problems that required Pythagorean Theorem in order to solve them.  Some examples were:

• Finding the length of the diagonal support board on a wooden gate
• Distance from one city to another (if we knew other lengths)
• TV sizes
• Distance from home plate to second base

Each of these real-world scenarios requires Pythagorean's Theorem to determine a missing length.

Click here for tonight's homework

March 16: Pythagorean Theorem Proof

Today we looked problems using Pythagorean Theorem and discussed more in depth how a² + b² = c².  Showing that this works every time is called a Proof.  The notes we did together in class are below:

Using the proof, we can determine missing side lengths if we know the area of the squares made by the sides.

Click here for tonight's homework.

March 12: Distance Formula

The Distance Formula is a rather ugly looking formula.  Almost terrifying in fact.  See?

 

More specifically, given the two points (x₁, y₁) and (x₂, y₂), the distance between these points is given by the formula above.


This is very similar to what we did yesterday when we found the distance using a graph.  No, really, it is!  Here- I'll prove it to you! Let's start with 2 arbitrary points: A and B.

We can run lines down from  A and B to create a right triangle (again exactly what we did yesterday.)  And we already know that a² + b² = c² thanks to Pythagoras.

 When we label the coordinates of points A and B, we can see the the intersection of the two legs we created has the same x-value as Point A and the same y-value as point B.

 So the length of side a is equal the change in x from point B to the intersection,

the length of side b is equal the change in y from point A to the intersection.

a = x₂ − x₁   and    b = y₂ − y₁

Click here for today's homework

March 11: Distance on a Graph

Today we learned how to determine distance between 2 points on a graph.

In order to determine the distance on a graph, we can use Pythagorean Theorem.  First we make a right triangle so that the distance we are looking for is the hypotenuse.  If we know the length of the legs (and because it's on a graph, we do!), we can easily solve for the missing side using a² + b² = c².

We did several examples in class and that sheet was secured in our math notebooks.

In each example, we drew a right triangle by using the segment given as the hypotenuse

.

Also, Progress Reports went out today, so please make sure those are SIGNED and RETURNED no later than FRIDAY, MARCH 13!  This is a homework assignment, so don't forget!

Click here for tonight's homework.

March 10: Converse of Pythagorean Theorem

Today we looked at the Converse of the Pythagorean Theorem.

Before we do that, let's consider what the Pythagorean Theorem is, because it's not JUST a² + b² = c²

The Pythagorean Theorem can be written as follows:

If a triangle IS a right triangle, then a² + b² = c².

The Converse states:

If a² +b² = c², then the triangle IS a right triangle.

Wait... isn't that the same thing?

Nope!  Think about it this way:

If it's the weekend, then we don't have school.  

What is the converse of that statement?

If we don't have school, then it's the weekend.

Is that true?  Not necessarily!  So the converse of the Pythagorean Theorem may at first seem like the exact same thing, but it really isn't!

We can use the converse to determine if a triangle is a right triangle.  If we know the side lengths, we put them into the formula and determine if the sides do in fact create a right triangle!

Click here for tonight's homework.  

March 9: Triangle Investigation

I was gone today, so our guest teacher had an investigation for Pre-Algebra today.  Students were to cut out squares of varying sizes, and use the sides of the squares to create triangles and record the data on the sheets given.

There are many relationships with triangle side lengths and the types of triangles that are created- specifically acute, obtuse, and right triangles.

Click HERE to manipulate a triangle and change it from acute to obtuse to right!

Tomorrow we will look at the relationships we noticed and see what conjectures we can make!

See you tomorrow!

March 6: Quiz Day!

Many students were gone today for a National Junior Honor Society field trip, so we had a pretty low key day.  We started with a time Perfect Squares and Perfect Cubes quiz, and then Intro to Pythagorean Theorem quiz after that.  If students were gone on Friday, they are required to retake the Intro to Pythagorean Theorem quiz, but they DO NOT have to take the Perfect Squares and Perfect Cubes quiz unless they choose to!

Next week we are going to delve into Pythagorean Theorem and see how it is used in the real world.

Have a great weekend!

March 5: Pythagorean Theorem

Today was the first day of Pythagorean Theorem.  We learned that the sum of the squares of the legs is equal to the square of the hypotenuse.  What?  Here's a better explanation with an example:

We saw a video proof of Pythagorean's Theorem as well.  When squares are created using the sides of a right triangle, we saw that when the wheel was turned over, the water in the two squares along the legs fill the square along the hypotenuse perfectly.

The equation for solving right triangles is 

a² + b² = c²

^{Click here for today's homework}

March 4: Equations with Square Roots

Today we learned how to solve equations by taking the square root.

We started with the equation a² + 17 = 20

Even though the variable has an exponent, we're going to pretend it doesn't.

So how would solve the simpler equation a + 17 = 20?  We'd start by isolating the variable.

Remember 2-Step Equations from AAALLLLLLLLL the way back in September?  That's exactly what this problem is, so that's exactly how we're going to solve it!

When we subtract 17 from both sides, we eliminate the constant on the left so that the term with the variable is the only thing on that side of the equal sign.

Now we know that

a² =4

Sooooooo, what number, when multiplied by itself, will equal 4?

If you're not sure, take the square root of each side to determine the answer!

 a = 2

Lastly, we need to substitute our answer in for the variable in the original problem to confirm our work.  Does a² + 17 = 20 when a = 2?

 

Yes, it works!  So we know that a = 2 is the correct answer.

 Click here for today's homework

March 3: Estimating Square Roots

Unit 6 Tests were returned today, so if you did not do as well as you would have liked, make sure you make your test corrections TONIGHT.  If your score was less than 70%, you are required to complete test corrections!

Today we talked about Estimating Square Roots.  In order to estimate the value of a square root of a number that is not a perfect square, it helps to first KNOW the prefect squares.

		12	=			1
		22	=			4
		32	=			9
		42	=			16
		52	=			25
		62	=			36

		72	=			49
		82	=			64
		92	=			81
		102	=			100
		112	=			121
		122	=			144

So if we are given the value √82, we can easily see the value will be between the integers 9 and 10, because the square root of 81 is 9, and the square root of 100 is 10.  And since 82 is much closer to 81 than it is to 100, we can also determine that the value of √82 is much closer to 9 than it is to 10.  To confirm this, we can check on a calculator.

And we can see that the square root of 82 is about 9.06, which is of course much closer to 9 than 10.

Click here for additional practice estimating square roots.

Click here for tonight's homework

And be sure to study your perfect square and perfect cube flashcards that were passed out today.  We will be taking a quiz on those on FRIDAY, MARCH 6.

March 2: Square Roots

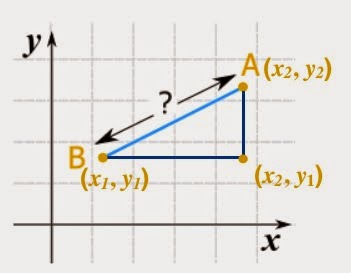
Happy Monday!  Today we started Unit 7: Square Roots and Pythagorean Theorem.

Today's lesson was an introduction to SQUARE ROOTS.  It's actually easier to start with SQUARES, and then talk about square roots.

To square a number, we just multiply it by itself.

For example, 5 squared = 5 × 5 = 25

When we take a number and multiply it by itself, we call the product a PERFECT SQUARE.

5 × 5 = 25

25 is a perfect square, because 5 × 5 = 25.  We can also say that 5 squared is 25.

Square roots go the other way: since 5 squared is 25, the SQUARE ROOT of 25 is 5.

A square root of a number is ...

... a value that can be multiplied by itself to give the original number.
 

A square root of 9 is ...

... 3, because when 3 is multiplied by itself we get 9.

It is like asking:

What can we multiply by itself to get this?

The first 12 squares:

1 Squared	=	12	=	1 × 1	=	1
2 Squared	=	22	=	2 × 2	=	4
3 Squared	=	32	=	3 × 3	=	9
4 Squared	=	42	=	4 × 4	=	16
5 Squared	=	52	=	5 × 5	=	25
6 Squared	=	62	=	6 × 6	=	36

7 Squared	=	72	=	7 ×7	=	49
8 Squared	=	82	=	8 ×8	=	64
9 Squared	=	92	=	9 × 9	=	81
10 Squared	=	102	=	10 ×10	=	100
11 Squared	=	112	=	11 ×11	=	121
12 Squared	=	122	=	12 ×12	=	144

Click here for today's homework